setrdigest.blogg.se

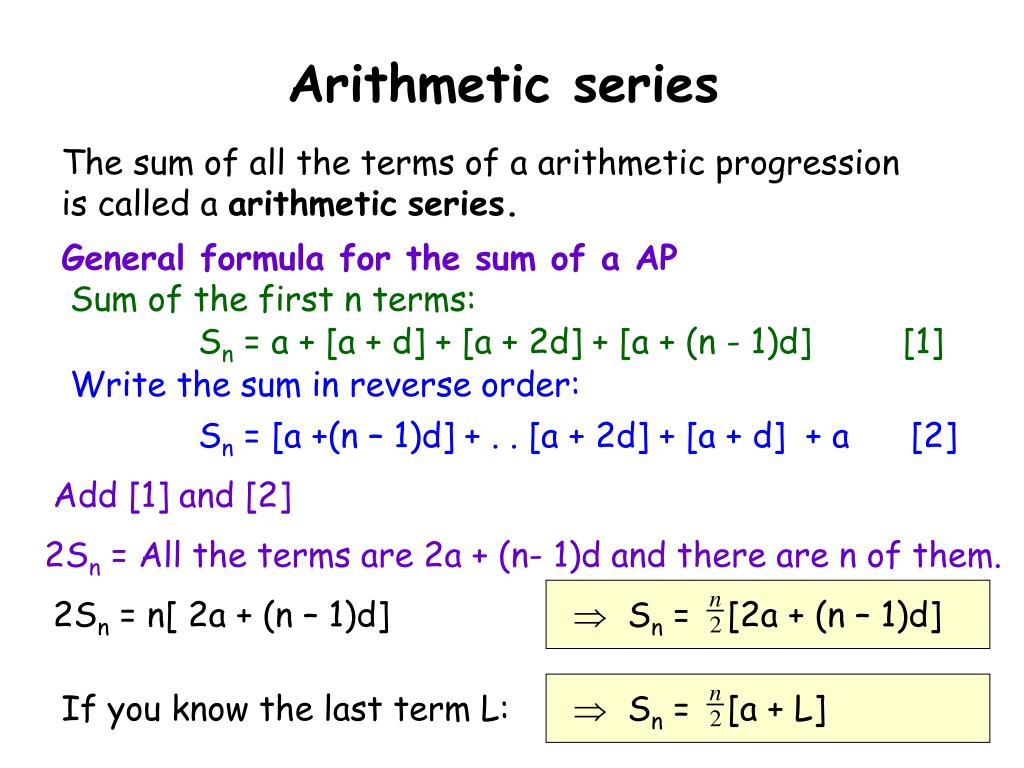
1. #Arithmetic and geometric sequences formulas sum plus#
2. #Arithmetic and geometric sequences formulas sum free#

If that interest is added every month, we say it is compounded monthly. This means the interest that was earned later gains its own interest. The interest on the account is calculated and added to the account at regular intervals. Compound interest is something that happens to money deposited into an account, be it savings or an individual retirement account, or IRA. Geometric sequences have a multitude of applications, one of which is compound interest. What is the sum of the first 6 terms of the geometric sequence with first term a 1 = 27 and common ratio r = 1 3? Using Geometric Sequences to Solve Real-World Applications Since each term is 1 2 1 2 times the previous, this is a geometric sequence. Each term is 1 2 1 2 times the previous term.

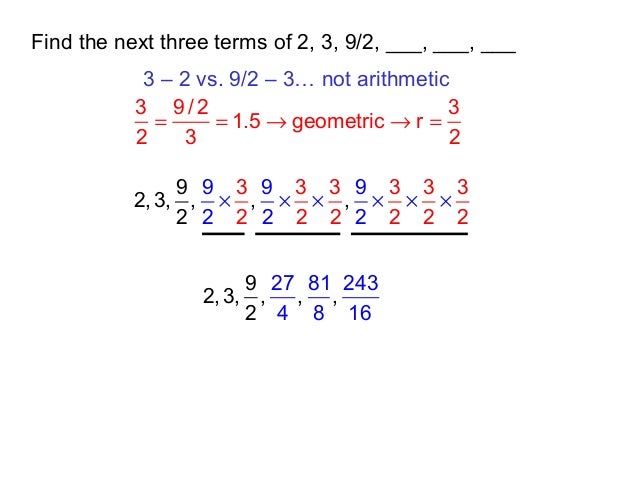
In the sequence, the change from 4 to 2 is a multiplication by 1 2 1 2, as is the next jump, from 2 to 1, as is the next from 1 to 1 2 1 2. In a geometric sequence, though, each term is the previous term multiplied by the same specified value, called the common ratio. So, you add a (possibly negative) number at each step.

#Arithmetic and geometric sequences formulas sum plus#

We know what a sequence is, but what makes a sequence a geometric sequence? In an arithmetic sequence, each term is the previous term plus the constant difference. This process exhibits exponential growth, an application of geometric sequences, which is explored in this section. Every 8 years, the investment would double again, so after the third 8-year period, the investment would be worth 2 × 2 × ( 2 × $ 400 ) = $ 1,600 2 × 2 × ( 2 × $ 400 ) = $ 1,600. After another 8 years (for a total of 16 years) the investment would be twice its value after the first 8 years, or 2 × ( 2 × $ 400 ) = 2 × ( $ 400 ) = $ 800 2 × ( 2 × $ 400 ) = 2 × ( $ 400 ) = $ 800. For example, if you invest $200 in an account with an 8-year doubling time, then in 8 years the value of the account will be double the starting amount, or 2 × $ 200 = $ 400 2 × $ 200 = $ 400. A shorter doubling times means the investment gets bigger, sooner. One of the concerns when investing is the doubling time, which is length of time it takes for the value of the investment to be twice, or double, that of its starting value.

Find the n nth term of a geometric sequence.Find a given term in a geometric sequence.– Suze Orman” by Morgan/Flickr, CC BY 2.0) Learning ObjectivesĪfter completing this section, you should be able to:

#Arithmetic and geometric sequences formulas sum free#

(credit: modification of “A big part of financial freedom is having your heart and mind free from worry about the what-ifs of life. Figure 3.49 Savings grows in a geometric sequence.